Newbie DIY speaker help required

[sunilraj]

Because baffle step rolls off at 6dB/octave till the transition point. Notice that F2 will *always* be double of F1, which is = one octave higher.

Basically:

1. A driver mounted on a baffle will show a 6dB increase in output at the baffle step frequency.

2. We need to counter this so that till that frequency, the output is 6dB higher.

3. We choose to use a second driver to offset this gain, by increasing the output below the baffle step frequency by 6dB.

4. Driver is connected in parallel to do this, but this results in the same output curve, only 6dB higher.

5. So we have to filter the frequencies so that the -6dB point of the 'lower' driver is = baffle step frequency (F2).

6. This will mean that the combination will output 6dB more in terms of raw response till the baffle step frequency, but once mounted on the baffle will produce a perfectly (within driver limits) linear response.

7. Since the -6dB point of a 6dB per octave crossover is at the crossover frequency and the rolloff perfectly matches (inversely) the baffle step curve, a 6dB/octave crossover works the best for this approach.

8. Therefore, we choose a 6dB/oct crossover with the XO frequency = F2 of the drivers on baffle.

Hope that clears it up. It's actually a very elegant solution.

Remember that a x.5 system (yours is 1.5) system is by nature inherently fully compensated for baffle step, so it may sound boomy in small rooms or if placed very close to the walls.

Cranky,
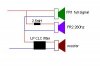
Please review below circuit. The CLC block ignores the reactances of parallel FR network. Please let me know your comments.

 

[sunilraj]

nononononono.........

You cannot integrate a woofer into a x.5 way setup unless you cross over again below that. The way you have planned it will turn the sound into mud.

You have two options:

1. Connect *only the two fullrange 8 inch speakers* with an inductor on the 'lower' woofer. There will be 2 speakers per channel for a 1.5 way setup.

2. Use one 8" speaker and one woofer per channel. The woofer will integrate the baffle step inductance into *its* crossover. Say you cross over at 300Hz, you will have the inductance of the required crossover frequency + the inductance of the baffle step correction in series with it. In this case, you have to put a high pass circuit on the 8" speaker to limit excursion and enhance power handling. You are free to choose the order and Fc of the crossover for your design.

It will happily take 30 watts or more if you cut off everything below 300Hz, and at 30 watts you will need to visit a doctor for a hearing aid soon after the listening session.

Edit: Also, the way you have it wired is extremely confusing. The lower woofer is in series with a parallel combination of the 8" speakers (ignoring the baffle step inductor for a minute). If you are planning a series crossover, that is not the way to implement it at all - look for series crossover diagrams, they are very different from the way you are seeing it.

Ok, Is there a way I can connect two FRs in Series (will not add woofer). Do a HF bypass capacitor will work instead of inductor.
I want to distribute available power between two instead of drawing more power from amp.